Description
What our Multiplying and Dividing Fractions lesson plan includes
Lesson Objectives and Overview: Multiplying and Dividing Fractions prepares students to multiply and divide fractions and mixed numbers. This lesson also demonstrates the use of factoring for multiplying and dividing fractions and mixed numbers. At the end of the lesson, students will be able to multiply and divide fractions and mixed numbers. The students will also be able to use factoring for multiplying and dividing fractions and mixed numbers. This lesson is for students in 4th grade, 5th grade, and 6th grade.
Classroom Procedure
Every lesson plan provides you with a classroom procedure page that outlines a step-by-step guide to follow. You do not have to follow the guide exactly. The guide helps you organize the lesson and details when to hand out worksheets. It also lists information in the blue box that you might find useful. You will find the lesson objectives, state standards, and number of class sessions the lesson should take to complete in this area. In addition, it describes the supplies you will need as well as what and how you need to prepare beforehand.
Options for Lesson
Included with this lesson is an “Options for Lesson” section that lists a number of suggestions for activities to add to the lesson or substitutions for the ones already in the lesson. Some of the optional adjustments to the lesson activity include using extra or less dice or a spinner to create the fractions for the activity. If you’d like to test your students’ understanding of the lesson material, you can use the practice page and/or homework page as a quiz or test. Finally, if you feel it’s needed, you can review basic multiplication and division facts with students prior to the start of the lesson.
Teacher Notes
The teacher notes page includes a paragraph with additional guidelines and things to think about as you begin to plan your lesson. This page also includes lines that you can use to add your own notes as you’re preparing for this lesson.
MULTIPLYING AND DIVIDING FRACTIONS LESSON PLAN CONTENT PAGES
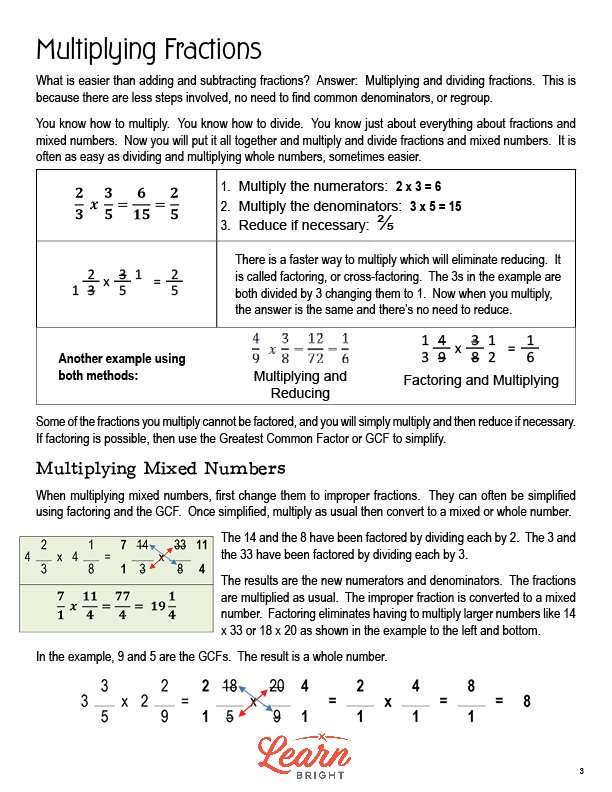
Multiplying Fractions
The Multiplying and Dividing Fractions lesson plan includes two content pages. Did you know that multiplying and dividing fractions is actually easier than adding and subtracting them? When multiplying and dividing, you don’t need to find common denominators or regroup!
To multiply fractions, you start by multiplying the numerators. Next, multiply the denominators. Finally, reduce if necessary. Let’s look at an example: 2/3 x 3/5. First, multiply the numerators: 2 x 3 = 6. Next, multiply the denominators: 3 x 5 = 15. Finally, put it together and reduce: 6/15 = 2/5.
There’s an even faster way to multiply, which will also eliminate the need to reduce. This method is called factoring (or cross-factoring). For the same example problem, you divide both 3s, changing them to 1s. You then multiply as normal to get the same answer without the need to reduce.
Let’s look at another example using both methods: 4/9 x 3/8. Using the first method, you first multiply the numerators: 4 x 3 = 12. Next, multiply the denominators: 9 x 8 = 72. Finally, reduce: 12/72 = 1/6. Using the second method, 4/9 x 3/8 becomes 1/3 x 1/2 = 1/6.
Sometimes, you’ll come across fractions that cannot be factored. In these cases, you can just multiply and reduce as usual. When factoring, use the Greatest Common Factor (GCF) to simplify.
Multiplying Mixed Numbers
To multiply mixed numbers, you first need to change them into improper fractions. You can then often simplify them using factoring and the GCF. Once you simplify, you can multiply as usual and convert to a mixed or whole number.
Let’s look at an example: 4 2/3 x 4 1/8. To start, you can factor the 14 and 8 by dividing each by 2 and factor the 3 and 33 by dividing each by 3. The results of this factoring are the new numerators and denominators: 7/1 x 11/4. You then multiply the fractions as usual and convert the mixed number to an improper fraction: 77/4 = 19 1/4. In this case, factoring eliminated the need to multiply large numbers.
Let’s look at another example: 3 3/5 x 2 2/9. For this problem, 9 and 5 are the GCFs and the final result is a whole number. When factored, the problem becomes 2/1 x 4/1 = 8.
Dividing Fractions
Dividing fractions is just as easy as multiply fractions! You just add one step. First, let’s review some vocabulary. When dividing whole numbers, such as 32 ÷ 8, 8 is the divisor. This is the number you divide by.
When dividing fractions, the first step is to invert, or flip, the divisor. The numerator becomes the denominator and the denominator becomes the numerator. For example, 2/3 becomes 3/2, 3/10 becomes 10/3, and 8/5 becomes 5/8.
Proper and improper fractions can both be inverted. If you’re dividing by mixed numbers, you first need to change the mixed number to an improper fraction before inverting it.
Always remember that you only invert the divisor. Once you’ve done that, all you need to do is multiply the fractions using the same methods described above.
Let’s look at an example: 2/3 ÷ 3/5. First, invert the divisor and change the problem to a multiplication problem: 2/3 x 5/3. Next, follow the usual multiplication steps: 2/3 x 5/3 = 10/9 = 1 1/9.
Let’s look at another example: 2 2/5 ÷ 3 1/3. First, change the mixed numbers to improper fractions and then invert the divisor before solving: 12/5 ÷ 10/3 = 12/5 x 3/10 = 36/50 = 18/25. In this example, you could have factored the 10 and 12 using 2 as the GCF if you wished.
Remember that, once you inver the divisor, the operation changes and it becomes a multiplication problem. You then follow the normal multiplication steps to solve. Most errors in fraction multiplication and division problems are computational errors so always pay attention and take your time!
The lesson closes with a few final tips. First, remember that whole numbers can be written as fractions by putting a 1 in the denominator. You can then invert them if needed. When multiplying or dividing fractions, ask these questions: Can I use a GCF before I multiply? Did I “flip” the right fraction? Can I reduce the answer or change it to a mixed or whole number?
If you cross-factor, use a small mark to cross out the numerator or denominator you divide into to help keep track. Finally, always stay neat and organized and check your work for errors.
MULTIPLYING AND DIVIDING FRACTIONS LESSON PLAN WORKSHEETS
The Multiplying and Dividing Fractions lesson plan includes three worksheets: an activity worksheet, a practice worksheet, and a homework assignment. You can refer to the guide on the classroom procedure page to determine when to hand out each worksheet.
DICE ACTIVITY WORKSHEET
Students will work with a partner to complete the activity worksheet. Each pair will build fraction multiplication and division problems by rolling three dice and adding the numbers together to use as their numerators and denominators. They will then solve each problem using the fraction multiplication and division rules, using factoring when possible. Finally, they will reduce or convert their answers if necessary.
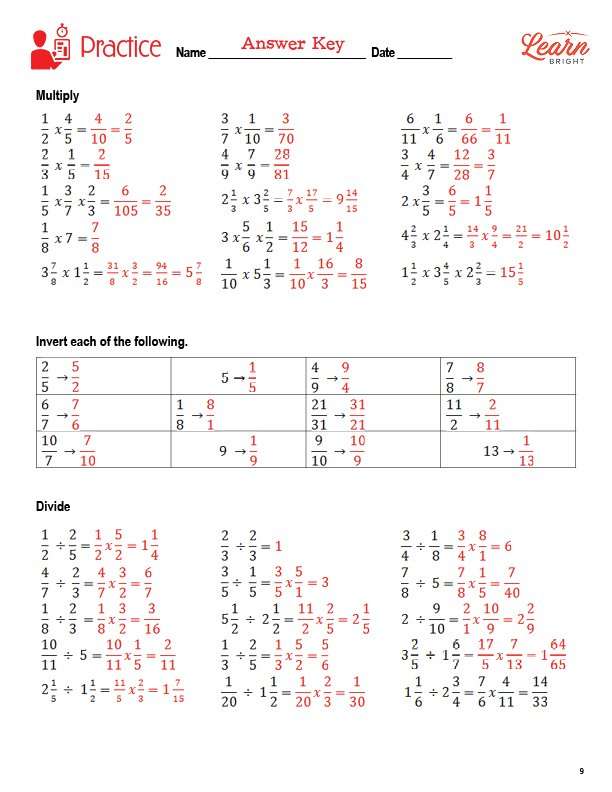
INVERT PRACTICE WORKSHEET
For the practice worksheet, students will solve multiplication and division problems that include fractions. They will also invert fractions and whole numbers.
MULTIPLYING AND DIVIDING FRACTIONS HOMEWORK ASSIGNMENT
The homework assignment asks students to solve ten word problems where they need to either multiply or divide fractions.
Worksheet Answer Keys
This lesson plan includes answer keys for the practice worksheet and the homework assignment. If you choose to administer the lesson pages to your students via PDF, you will need to save a new file that omits these pages. Otherwise, you can simply print out the applicable pages and keep these as reference for yourself when grading assignments.