Description
What our GCF and LCM lesson plan includes
Lesson Objectives and Overview: GCF and LCM teaches students about Greatest Common Factors and Least Common Multiples, reviews all related vocabulary and offers multiple practice examples for teachers to work through with their students. At the end of the lesson, students will be able to find the greatest common factor and least common multiple using multiplication facts and prime factorization. This lesson is for students in 6th grade.
Classroom Procedure
Every lesson plan provides you with a classroom procedure page that outlines a step-by-step guide to follow. You do not have to follow the guide exactly. The guide helps you organize the lesson and details when to hand out worksheets. It also lists information in the blue box that you might find useful. You will find the lesson objectives, state standards, and number of class sessions the lesson should take to complete in this area. In addition, it describes the supplies you will need as well as what and how you need to prepare beforehand.
Options for Lesson
Included with this lesson is an “Options for Lesson” section that lists a number of suggestions for activities to add to the lesson or substitutions for the ones already in the lesson. One optional addition to this lesson is to have the students practice problems with a partner in the class. You can also use whiteboards to show multiples and practice prime factorization. Another option is to use manipulatives in the classroom to break apart numbers. Finally, you may want to review skip counting before teaching the lesson.
Teacher Notes
The teacher notes page includes lines that you can use to add your own notes as you’re preparing for this lesson.
GCF AND LCM LESSON PLAN CONTENT PAGES
Greatest Common Factor
The GCF and LCM lesson plan includes three content pages. The greatest common factor, or GCF, of two numbers is the largest factor that will divide into both numbers. Factoring is when you take a number apart using multiplication. Factors are the numbers that are multiplied together when factoring. Common factors are the factors that are the same for both numbers. Two numbers can have one or many common factors. The greatest common factor is the largest factor that the numbers have in common.
We can find the greatest common factor in two ways. First, we can list all of the factors of a number by thinking of all the multiplication facts for that number. For example, to find the GCF of 15 and 9, we first list out their factors. The The factors of 15 are 1, 3, 5, and 15, because 1 x 15 = 15 and 3 x 5 = 15. The factors of 9 are 1, 3, and 9, because 1 x 9 = 9 and 3 x 3 = 9. We then identify any factors that are the same for 15 and 9. 1 and 3 are the common factors of 15 and 9. 3 is larger than 1, so the greatest common factor is 3.
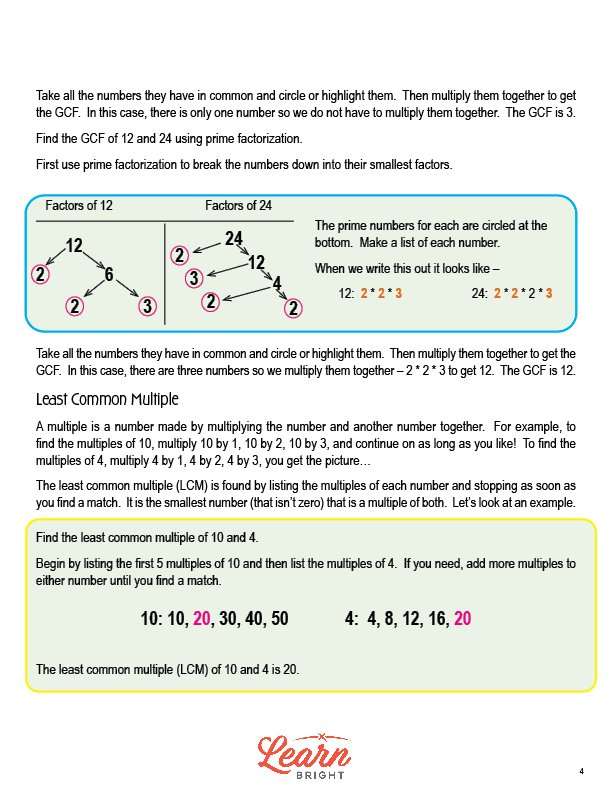
Sometimes, finding all of the multiplication facts for a number is too difficult, especially for a large number. In these cases, we can use prime factorization instead. This is the second way to find the GCF. To to find the GCF of 15 and 9 using prime factorization, we first break the numbers down into their smallest factors. For 15, these are 3 and 5. For 9, these are 3 and 3. Next, we look at any numbers they have in common and multiply them together to get the GCF. In this case, there’s only one number in common so we don’t have to multiply them together. The GCF is 3.
Let’s look at an example where you do need to multiply the numbers together at the end. To find the GCF of 12 and 24 using prime factorization, we first break the numbers down into their smallest factors. For 12, these are 2, 2, and 3. For 24, these are 2, 2, 2, and 3. We identify the numbers they have in common and multiply them together: 2 x 2 x 3 = 12. 12 is the GCF!
Least Common Multiple
Multiples are numbers that we make by multiplying two numbers together. To find the multiples of 10, for example, we can multiply 10 x 1, 10 x 2, 10 x 3, and so on. We find the least common multiple (LCM) by listing the multiples of each number and stopping once you find your first match. The LCM is the smallest number (other than zero) that is a multiple of both numbers.
For example, to find the LCM of 10 and 4, we start by listing the first five multiples of 10 and 4, adding more as needed until we find a match. The multiples of 10 are 10, 20, 30, 40, and 50. The multiples of 4 are 4, 8, 12, 16, and 20. Looking at the lists, we can see that the LCM of 10 and 4 is 20.
Remember that your first step is to make a list of multiples of each whole number, continuing until you find a match. The first match is the LCM.
GCF AND LCM LESSON PLAN WORKSHEETS
The GCF and LCM lesson plan includes four worksheets: an activity worksheet, a practice worksheet, a homework assignment, and a quiz. You can refer to the guide on the classroom procedure page to determine when to hand out each worksheet.
CALENDAR ACTIVITY WORKSHEET
The activity worksheet asks students to look at printed calendars and circle the dates for each month that match the stories that the teacher tells. For example, for July, they will circle the dates on the calendar in which they’ll get to eat ice cream twice if an ice cream truck drives through their neighborhood every third day and their best friend’s neighborhood every fifth day.
GCF AND LCM PRACTICE WORKSHEET
For the practice worksheet, students will first find the GCF of two sets of numbers using either the multiplication facts or prime factorization methods. Next, they will find the LCM of three sets of numbers.
STORIES HOMEWORK ASSIGNMENT
The homework assignment asks students to create their own stories like the one from the lesson activity. They will write three short stories for three different calendar months. They will decorate their calendars to match their stories and will share their stories with the class.
QUIZ
This lesson also includes a quiz that you can use to test students’ understanding of the lesson material. For the quiz, students will first find the GCF of two numbers. They will then find the LCM of two other numbers.
Worksheet Answer Keys
This lesson plan includes answer keys for the practice worksheet and the quiz. If you choose to administer the lesson pages to your students via PDF, you will need to save a new file that omits these pages. Otherwise, you can simply print out the applicable pages and keep these as reference for yourself when grading assignments.